50+ IB Maths AA IA Ideas
check out our results
Request Free Trial Class
This list contains a selection of curated IB Math AA IA ideas It includes 50+ specific ideas for IB Math IA Internal Assessment Examples and topics. The topics cover a wide range of mathematical concepts, including calculus, algebra, geometry, statistics, and probability, and are suitable for both SL and HL students and it also includes MATHS AA IA samples.
IB Math AA HL IA Ideas
1.) The Math of Infectious Disease: Understanding and Interpreting Models in Epidemiology
Mathematics:
Using mathematical concepts like exponential functions and calculus to derive differential equations from various epidemiological models like SARS, etc.
Procedure:
In order to proceed with this topic, you must derive differential equations based on the model and find the solution using various methods like the homogenous method, linear method, etc.
Analysis:
The derivation of such differential equations for epidemiological models are of great importance when it comes to analyzing trends and forecasting data. The information gathered can then be used to control the spread of the disease and prevent future outbreaks.
2.) Investigating the mathematical harmony of Beethoven
Mathematics:
If you’re a classical music geek, this one’s for you. There are various mathematical properties that surround music such as trigonometry.
Procedure:
In the case of Beethoven, you can graph the notes and chords with sine waves. The simplest model of a musical sound is a sine wave, in which the domain (x-axis) is time and the range (y-axis) is pressure.
Analysis:
Using such mathematical concepts helps strengthen knowledge in music as counting, rhythm, scales, intervals, patterns, symbols, harmonies, time signatures, tone and pitch are determined by using math.
3.) Understanding China’s Income Distribution from a Mathematical Perspective using the Lorenz Curve and Gini Coefficient
Mathematics:
Using various mathematical concepts like integration and functions.
China’s income distribution can be found using secondary methods or primary, depending on your geographical location.
Procedure:
Using the data, you can plot Lorenz’s curve using Gini Coefficient to show the ideal income inequality in an economy as well as the reality. The areas below the graph using integration and the difference between the two will depict the gap between the rich and the poor.
Analysis:
Such models are of great importance when it comes to analysing trends and forecasting data. The Lorenz curve is important because it helps in understanding economic inequality. When the lorenz curve keeps moving away from the baseline it indicates that the level of unequal distribution keeps increasing.
4.) Investigating the safe operating speed for ground vehicles on a given road segment
Mathematics:
Using mathematical concepts such as integration when calculating area under the graph and finding optimum speed. When driving a car, it is often necessary to slow down during a curve. This optimum speed can be found using mathematics.
Procedure:
An observer is placed at each end of the survey segment recording each vehicle’s passing time and license plate, in order to calculate the time spent traversing the segment. This data can be used to derive the formula for speed and differentiate it in order to find maximum and minimum points.
Analysis:
The optimum speed when travelling in a curve is found for various reasons, the most important one being safety. Determining the optimum speed to operate at a turning is absolutely crucial when it comes to safety.
5.) Calculating orbital flight paths/intercept trajectories in space for rockets
Mathematics:
Using mathematical concepts such as differentiation and integration to calculate the orbital flight path.
Procedure:
You will have to graph the pathway in which the rocket will move as well as model the rocket to determine the optimum speed and path.
Analysis:
Such analysis is crucial in order to reduce the fuel efficiency of rockets in space using gravity assist.
6.) Using origami to design foldable furniture
Mathematics:
There is plenty of math’s related to origami. Origami works on math principles and theorems. One can apply algebra, sequences and series, calculus and logical ability to design foldable furniture analogous to folding paper in origami.
Procedure:
You will have to pick a tessellation which you will use to design the piece of furniture and then decide the optimum number of folds (using calculus) for the size of furniture you’ve picked
Analysis:
Designing a foldable furniture is useful when there are small areas. For example, folding a dining table into a coffee table. Applying Origami and math to it would help to determine the most optimized way of doing the same.
7.) Numerical model of the solar system
Mathematics:
The planets orbit around the sun in elliptical paths, with sun at one of the foci. Using geometry and algebra you can find the equations of each planets and model the entire solar system.
Procedure:
Using data from the web you can find the equations using the concept of ellipses. As an extension you can find the areas that each planets covers as they revolve around the sun and compare their properties that are affected due to this revolution.
Analysis:
If you’re interested in space, this exploration will give you insights of planetary motion and how math is involved in understand the physical world.
8.) Analysis of AC circuits by using complex numbers
Mathematics:
If you’re a physics student and wish to dig deeper in math’s behind AC circuits, you can start by applying complex numbers to the previously leant concepts.
Procedure:
Complex numbers are utilized in calculations of current, voltage or resistance in AC circuits. One can make use of math to analyze AC circuits.
Analysis:
Math’s and Physics go hand in hand. Physics makes use of mathematical tools to justify and understand the theorems and concepts. This exploration will help understand the relation between math’s and physics.
9.) Chess and Mathematics
Mathematics:
Combinatory is the mathematics of counting and probabilities. You can explore chess and all the different ways a chess game can play out using statistics, probability and combinatory.
Procedure:
Math exists inherently in the game. Starting with the initial positions of the chess pieces, start to analyses all the possible moves and use mathematical tools to figure various ways in which the game can be played out. Instead of dealing with the whole game, you can also create situations on the board which a player might face and then using math calculate all the possible moves and find out the right one.
Analysis:
This exploration connects the game of chess with math. Although there will be numerous simulations of the game possible, one can still analyses the certain situations on the board using math’s and make conclusions.
10.) Mathematics of solar panel
Mathematics:
Solar energy has great importance in today’s world. There are various mathematical tools that can be used to design and install the most optimum solar panel. This exploration may involve vectors, trigonometry and calculus.
Procedure:
The various aspects which you can work on are-
- Optimum tilt of the solar panel
- Find the size of PV modules according to use
- Number of PV panels required
Analysis:
Solar panels are installed in many households and according to the power consumption, they must be customized. This exploration makes use of math to determine the most optimal way to make use of solar panels.
11.) Relation of diversity of ecosystem and climate
Mathematics:
Referring to previous techniques in mapping the relation and implementing them in sample data such as diversity indices and parameterization along with providing effective numbers
Procedure:
Obtain data of diversity and climatic conditions for a variety of regions with different properties and implement diversity indices and parameterization.
Analysis:
The data and mathematics will help determine the diversity of species that survive and require the variety of climatic conditions
12.) Relationship between number of gear shifts and probability to win in a F1 race
Mathematics:
Utilising previous statistics of F1 races and probability, we determine how drivers who have won shift the gears of their cars along with its frequency
Procedure:
Gather previous race statistics of different positioned racers in one or more series of races while noting the car model
Analysis:
F1 is an exhilarating sport and victories are determined not only by the car’s performance but also the decisiveness of the driver, which includes the number and pace of gear shifts throughout the track.
13.) The Golden Chord Progression
Mathematics:
Utilising the golden ratio to determine harmonious and discordant chords
Procedure:
The golden ratio can be applied to sample melodies and songs which can provide a progression series of chords.
Analysis:
The golden chord progression is a technique that enables music producers to determine chord progressions that fit a melody based on the melody’s properties.
14.) Investigating the mathematics of cryptography
Mathematics:
Cryptography involves the use of mathematical algorithms and techniques to secure information and protect it from unauthorised access.
Procedure:
One way to investigate the mathematics of cryptography is to examine different encryption and decryption methods, such as the RSA algorithm and the Diffie-Hellman key exchange protocol. This can involve analysing the mathematical properties of these methods and exploring how they are used in real-world applications.
Analysis:
By studying the mathematics of cryptography, one can gain a deeper understanding of how information is secured and how cryptographic techniques can be used to protect sensitive data. This can lead to new insights and discoveries in the field of cryptography, as well as in related areas such as computer science and cybersecurity.
15.) Investigating the mathematics of chaos theory
Mathematics:
Chaos theory is a branch of mathematics that studies the behaviour of dynamical systems that are highly sensitive to initial conditions.
Procedure:
One way to investigate the mathematics of chaos theory is to explore the properties of chaotic systems, such as the Lorenz system or the logistic map. This can involve analysing the bifurcation diagrams and phase portraits of these systems, as well as studying the Lyapunov exponents and other quantitative measures of chaos.
Analysis:
By studying the mathematics of chaos theory, one can gain a better understanding of the complex and often unpredictable behaviour of natural systems. This can lead to new insights and discoveries in fields such as physics, meteorology, and biology, as well as in the development of new mathematical models and computational techniques.
16.) Optimization of a Manufacturing Process
Mathematics:
Use linear programming to create a mathematical model that represents the manufacturing process. You could use linear programming to optimize a manufacturing process, finding the optimal allocation of resources to maximize efficiency or minimize costs.
Procedure:
The optimization of a manufacturing process involves defining the problem, formulating a mathematical model using linear programming, solving the model to find the optimal solution, interpreting the results, and implementing the solution.
Analysis:
This allows you to find the optimal allocation of resources to maximize efficiency or minimize costs.
17.) Optimizing the Design of a Roller Coaster
Mathematics:
This IA topic involves a range of calculus concepts, including differentiation, integration, optimization, and modeling
Procedure:
Modeling the Roller Coaster: Use calculus to model the path of the roller coaster, including the height, speed, and curvature of the track. Optimizing the Path: Use optimization techniques such as the derivative and the second derivative to optimize the design of the roller coaster, such as minimizing the maximum speed or maximizing the vertical drop.
Analysis:
This topic involves using calculus to model the path of a roller coaster, and optimizing the design to maximize the thrill factor while ensuring the safety of the riders.
18.) Approximating Trigonometric Functions using Maclaurin Series
Mathematics:
This topic involves exploring the properties and application of Maclaurin series to approximate various trigonometric functions.
Procedure:
Discuss the properties of Maclaurin series and derive the series expansions for various trigonometric functions, such as sine, cosine, and tangent. Analyze the accuracy of the Maclaurin series approximations for different ranges of x-values and determine the number of terms needed to achieve a certain level of accuracy.
Analysis:
Explore the applications of Maclaurin series approximations in various fields, such as physics, engineering, and finance, and discuss their limitations and practical considerations.
19.) Optimization Problems in Economics
Mathematics:
This topic involves applying the Lagrange multiplier method to solve optimization problems in economics.
Procedure:
Identify a specific optimization problem in economics, such as maximizing profit, minimizing cost, or optimizing utility subject to certain constraints. Apply the Lagrange multiplier method to the chosen optimization problem, analyze the results, and interpret the implications in the context of the economic problem.
Analysis:
To stay competitive by generating higher value for customers, businesses must optimize profit, cost and utility.
20.) Investigating the Mathematics of Predator-Prey Models
Mathematics:
This topic involves an investigation into the mathematical models used to describe the dynamics of predator-prey relationships in an ecosystem. The IA would involve developing a set of differential equations to model the interactions between predator and prey populations, using techniques such as Lotka-Volterra equations.
Procedure:
The research could explore the conditions for equilibrium and stability of the system, as well as the effects of various factors on the dynamics of the system, such as the initial populations, the rates of predation and reproduction, and environmental factors.
Analysis:
The IA could include numerical simulations, graphical analysis, and sensitivity analysis to draw conclusions about the behavior of the system and its practical applications in ecology and conservation biology. Additionally, the IA could explore the limitations and assumptions of these models and propose ways to improve them.
21.) Analyzing Population Growth using Differential Equations
Mathematics:
This topic involves an investigation into the use of differential equations to model and analyze population growth.
Procedure:
The IA would involve selecting a population to model, such as a species of animal, and using mathematical techniques to construct and analyze a differential equation that represents the population dynamics. The research could explore various factors that influence population growth, such as birth and death rates, carrying capacity, and environmental factors, and how they can be incorporated into the differential equation model.
Analysis:
The IA could include simulations, graphical analysis, and numerical solutions to draw insights and conclusions about the population dynamics and how they change over time. The research could also consider the implications of the results for conservation efforts or policy decisions related to the population in question.
22.) Analyzing the Stability of Nonlinear Systems using Isoclines
Mathematics:
This topic involves an investigation into the use of isoclines to study the stability of nonlinear systems, such as differential equations or discrete maps.
Procedure:
The IA would involve a comparison of various isocline-based methods, such as phase portraits, equilibrium analysis, and bifurcation diagrams, using mathematical techniques to analyze their effectiveness and accuracy. The research could also explore the influence of key factors on the stability of these systems, such as system parameters, initial conditions, and external perturbations.
Analysis:
The IA could include simulations, sensitivity analysis, and stability analysis to draw conclusions about the relative effectiveness of these methods and their practical applications.
Download our Successful College Application Guide
Our Guide is written by counselors from Cambridge University for colleges like MIT and other Ivy League colleges.
To join our college counseling program, call at +918825012255
IB Math AA SL IA Ideas
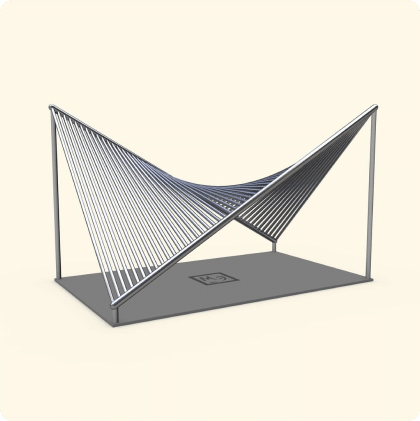
1.) Surface Area of Hyperbolic Paraboloid – Integration by substitution
Mathematics:
Differentiation and integration are the most dominant mathematical concepts when it comes to calculating the surface area of a hyperbolic paraboloid.
Procedure:
Pick an object with a hyperbolic paraboloid and then plot it on a graph. Then you must split it into different parts. Derive the equation. Calculate the surface area using integration.
Analysis:
This analysis is most important when it comes to a business’s point of view. For instance, when manufacturing products of a hyperbolic paraboloid, one must optimise space to ensure consumer satisfaction.
2.) Modelling the shape of an egg
Mathematics:
The are various mathematical concepts when modelling the shape of an egg, one of the most important ones being integration.
Procedure:
Firstly, you would have to draw the egg and then plot it on a graph. Then you must derive the equation. Calculate volume and surface area using integration.
Analysis:
The geometrical properties of eggs – such as volume and surface area – have uses ranging from ecological, physiological and morphological studies in birds, to predictions of conditions in the poultry industry.
3.) The correlation between fertiliser concentration and plant growth
Mathematics:
Using mathematical concepts like Pearson’s correlation. This is a statistical tool that tests the strength of a linear correlation.
Procedure:
If you are a biology student, then this topic would be apt for you. Firstly, you must take at least 5 different pots with soil and a seed sown into it. Next, add varied concentration of fertilizer to each pot, as well as a control experiment. Measure plant growth over the span of 15 days (depending on the seed). Collect this data and use Pearson’s correlation to determine how strongly correlated the two variables are.
Analysis:
This research is especially important in finding out if the fertilizer has a significant impact on plant growth, as well as to find the optimum concentration
4.) Modelling the pharmacokinetic profile of erythromycin
Mathematics:
Using mathematical concepts such as exponential functions and integration. The pharmacokinetic profile is the way and the amount of time in which a drug moves and gets absorbed by your body.
Procedure:
In this case, you would have to collect secondary data. Using the data points, the half-life of erythromycin can be calculated. Depending on the result, you can figure out at which stage is the drug most active.
Analysis:
It is highly important to know at which stage the drug is most active from the consumer’s and producer’s perspectives in order to determine at what time to consume the medicine and how long its effects will last.
5.) Modeling a cooling cup of tea
Mathematics:
This IA is about finding the time it takes for a cup of tea to cool down using exponential functions.
Procedure:
To gather the data I needed to produce the graph a cup of tea cooling down needs to be measured. Using a temperature sensor and piece of graphing software a set of readings that measured the temperature of the tea can be produced. 3 exponential equations are derived with graphs and separate sets of data.
Analysis:
By comparing the average error difference of all 3 equations, one equation will be narrowed down. Using this equation, the time for the tea to cool down is calculated.
6.) Is there a correlation between hours of study and exam grades?
Mathematics:
Using bivariate statistics, one can find correlation between any two things that seem to be affecting one another. It would involve Pearson moment correlation coefficient and other statistical methods.
Procedure:
You will have to collect data for the same and make a scatter plot. Further calculate the required statistics and make the conclusions. Further exploration may include modelling of the data using various other functions.
Analysis:
There are various types of students, some work less and are still able to score well because of efficient work, and some are opposite. Does this mean the there is no relation between studying hours and grades? Using mathematical tools one can try to make a conclusion with the data collected from around them.
7.) Optimization of profit of a start up
Mathematics:
Calculus can be used to maximize profit in a business. Along with that Algebra can be used to find breakeven points, so that a person can work accordingly towards the profit goals.
Procedure:
First thing is to set up different expenses involved in a business and optimize all of them using calculus. Calculate the breakeven point. Further calculate the profit equation and optimize it.
Analysis:
This exploration will help you understand the various factors involved in a new business and the things that need to be taken care of. The math application to a start-up gives an insight on how you can build a business while considering every factor and planning profits for the long run.
8.) Weaving a spider web
Mathematics:
If you’ve every observed a spider web, you must have seen geometric patterns and symmetry. One can also make use of algebra and calculus to form a spider web.
Procedure:
Taking the center of the spider web to be at the origin, one can use Cartesian and polar coordinates to determine the equations of the entire web. Use of trigonometric functions will also be involved.
Analysis:
This exploration will help model real life scenarios by taking the example of a spider web. Also it will enable us to understand that how nature forms geometric and symmetric patterns around us with so much accuracy.
9.) Photography and math
Mathematics:
If you’re interested in photography, this could be your chance to explore math behind your camera. Geometric sequences, geometry and some Algebra will be used for this exploration.
Procedure:
The shutter speeds of camera form a geometric sequence, which in turn is related to the aperture of the lens. This affects the brightness of the pictures taken. Another aspect that can be explored is the rule of thirds which is used by photographers to take pictures, which comes from the well-known golden ratio.
Analysis:
This exploration will enable us to understand that how art and math are related. The working of a camera is mostly based on math.
10.) Analysis of projectile motion on different planets (Varying gravity)
Mathematics:
Projectile motion is modelled by quadratic equations. The path depends on the gravity, the angle of projectile and the initial speed.
Procedure:
The best way to go around this would be by using parametric equations for the projectile (Quadratics can also be used). Consider planets, the sun or even black holes for the analysis.
Analysis:
This exploration will give a great idea about how gravity affects life on various different kinds of bodies in space (hypothetically of course!).
11.) Optimization of Packaging Materials
Mathematics:
The theory of optimization to calculate the optimal packaging required for common products with cylindrical, cuboidal and conical shapes.
Procedure:
Derive the formulas for these shapes so that they have the least surface area and utilize minimum packaging materials. Using the formula, common products will be assessed on how much packaging materials are wasted in their production.
Analysis:
Using the calculations, evaluate different industrial products (conical in shape, cylindrically shaped, cuboidal products) to determine the optimality of their packaging methods.
12.) Approximation of Phi
Mathematics:
This investigation aims to explore the famous golden ratio known as Phi using various mathematical approaches like Trigonometry, Geometry (Line Segments), Quadratic Equation, Continued Fraction, Integration and Fibonacci Sequence.
Procedure:
- Line Segment – Draw a line segment and divide it into two segments. Each segment will result in a different ratio for the smaller line segment to the larger line segment, and of the larger line segment to the whole line segment.
- Quadratic Equation – Consider the two line segments and rearrange the equation which can be solved using quadratic formula.
- Fibonacci Sequence – Make a sequence by adding two previous numbers in succession starting with 0 and 1.
Analysis:
- Line Segment – Golden Ratio is obtained when two segments when two segment lengths have the same proportion as the proportion of their sum to the larger of the two lengths.
- Quadratic Equation – There will be two results obtained. However, the positive value will be the approximation to the phi value.
- Fibonacci Sequence – The ratio of two consecutive numbers in the sequence will approximate to Phi value.
13.) Exploration of Euler’s Number “e”
Mathematics:
This investigation aims to explore the Euler’s number “e”. Using multiple mathematical concepts to derive, calculate and approximate “e”. These include: probability, limits, sequence and series, continued fractions, and calculus.
Procedure:
Using the simple interest formula, the number of times the principal amount is compounded (n) derives the final amount after interest.
- Limits – Consider a positive integer, integrate the equation and then solve the integrals.
- Sequence and Series – Represent the exponent function by a series with coefficients that are unknown and then apply the property of the derivative of the exponent function. To calculate “e” substitute different values of k in the equation.
Analysis:
As the value of n increases the value of S (accumulated amount) comes closer to that of e. As n tends to infinity, S converges to “e”.
- Limits – Using graphs, plot the equation. From the graph it will be evident that there is a horizontal asymptote. Evidently, this asymptote is the line y = e.
- Sequence and Series – As the value of k increases the value of sum of the series will get closer to “e”.
14.) Investigating Josephus Problem
Mathematics:
In mathematics, the josephus problem is a theoretical problem associated with a specific counting-out game. RQ: To figure out which position to stand in, in order to be the last man standing by creating and applying different formulas through Josephus Problem.
Procedure:
To find out the Josephus problem, i.e. to figure out the position to stand in, in order to be the only man remaining in the circle by using different mathematical applications such as pattern recognition, solving problems using power of two. Also, derive a general formula for the Josephus problem and calculate the winning position for the Josephus problem.
Analysis:
Two patterns will be derived. In the first pattern all the winning positions were odd and in the second pattern the winning position is jumping by two numbers each time and resists at one at some point that is when a number is a power of two.
15.) Discovering patterns within Pascal’s Triangle
Mathematics:
One of the most interesting number patterns in Pascal’s Triangle.
Procedure:
In order to build the triangle, start with 1 at the top, then continue placing numbers below it in a triangular pattern. Each number is the numbers directly above it added together.
Analysis:
- First Diagonal will have just 1’s
- Second Diagonal will have the counting numbers (1,2.3. etc)
- Third Diagonal will have the triangular numbers
- Fourth Diagonal will have the tetrahedral numbers
- The triangle will be symmetrical
- Horizontal sums double each time (Power of 2)
- Each line is the exponent of 11.
16.) Derivation of Kepler’s Law
Mathematics:
Deriving Kepler’s Law utilising the principles of polar coordinates and calculus
Procedure:
Research the history of Kepler’s Law and implement the principle of polar coordinates and calculus to obtain the equations produced by the law in accordance to other principles and assumptions
Analysis:
Kepler’s law brought light on the movement and rotation of objects in the solar system and explains the positioning of the planets and moon.
17.) Modelling the Surface Area of Jello
Mathematics:
Calculating the surface area of jello using modelling techniques and tools along with calculus.
Procedure:
Modelling tools and techniques help provide equations that can also be correlated and compared to calculus equations that determine the surface area of the jello.
Analysis:
Modelling the surface area of jello is important in its manufacturing as it determines the ideal way to consume it while ensuring that customers are satisfied
18.) Investigating the distribution of prime numbers
Mathematics:
Prime numbers have always been fascinating in the world of mathematics. Investigating the distribution of primes can involve the use of concepts such as number theory, probability, and statistics.
Procedure:
One way to investigate the distribution of prime numbers is to use a sieve method such as the Sieve of Eratosthenes. This involves crossing out all multiples of primes up to a certain limit, which leaves the remaining numbers as potential primes. Another approach is to use the Riemann hypothesis, which relates the distribution of primes to the distribution of zeros of the Riemann zeta function.
Analysis:
By analysing the distribution of prime numbers, one can gain a better understanding of the patterns and relationships that exist within the set of prime numbers. This can lead to new insights and discoveries in the field of number theory.
19.) Investigating the mathematics of origami
Mathematics:
Origami, the art of paper folding, involves many mathematical concepts such as geometry, symmetry, and topology.
Procedure:
One way to investigate the mathematics of origami is to examine the various types of folds and how they affect the resulting shape of the paper. For example, crease patterns can be analysed to determine the angles and lengths of the folds, which can then be used to calculate the resulting shape.
Analysis:
By studying the mathematics of origami, one can gain a better understanding of the underlying principles that govern the art of paper folding. This can lead to new techniques and methods for creating complex origami designs, as well as insights into other areas of mathematics and science that are related to origami, such as topology and graph theory.
20.) Investigating the mathematics of game theory
Mathematics:
Game theory is a branch of mathematics that studies the strategic interactions between decision-makers.
Procedure:
One way to investigate the mathematics of game theory is to analyse various types of games, such as the prisoner’s dilemma or the game of chicken. This can involve studying the payoff matrices and equilibrium solutions of these games, as well as exploring the various strategies and tactics that players can use to maximise their outcomes.
Analysis:
By studying the mathematics of game theory, one can gain a better understanding of how strategic decision-making works in various contexts, such as business, politics, and economics. This can lead to new insights and discoveries in the field of game theory, as well as in related areas such as decision theory and behavioural economics.
21.) Modelling the Surface Area of Jello
Mathematics:
Calculating the surface area of jello using modelling techniques and tools along with calculus.
Procedure:
Modelling tools and techniques help provide equations that can also be correlated and compared to calculus equations that determine the surface area of the jello.
Analysis:
Modelling the surface area of jello is important in its manufacturing as it determines the ideal way to consume it while ensuring that customers are satisfied
22.) Modeling the Spread of a Contagious Disease
Mathematics:
This topic involves an investigation into the use of mathematical models to study the spread of a contagious disease, such as COVID-19.
Procedure:
The IA would involve collecting data on the number of cases and deaths, as well as other relevant variables such as population density and mobility, and then using mathematical techniques to create and analyze models of disease spread. The research could also explore the influence of key factors on the behavior of the disease, such as vaccination rates, social distancing measures, and testing strategies.
Analysis:
The IA could include simulations, sensitivity analysis, and validation testing to draw conclusions about the effectiveness and practicality of different modeling approaches. This topic could also involve exploring the implications of different modeling scenarios on public health policy and decision-making.
23.) Calculating the Volume of a Complex Solid using Integration
Mathematics:
This topic involves an investigation into the use of integration to calculate the volume of a complex solid, such as a three-dimensional shape with irregular boundaries.
Procedure:
The IA would involve a comparison of various integration-based methods, such as disks and shells, using mathematical techniques to analyze their effectiveness and accuracy. The research could also explore the influence of key factors on the accuracy of these methods, such as the complexity of the solid, the precision of the measurements, and the numerical methods used to perform the integration.
Analysis:
The IA could include graphical representation, limits, and Riemann sums to draw conclusions about the relative effectiveness of these methods and their practical applications.
24.) Calculating the Length and Area of a Roller Coaster using Parametric Equations
Mathematics:
This topic involves an investigation into the use of parametric equations to calculate the length and area of a roller coaster track, which is a complex curve with changing curvature and elevation, using graphical representation, derivatives, and integrals.
Procedure:
The IA would involve developing parametric equations that describe the roller coaster track, and then using mathematical techniques to analyze their properties and behavior. The research could also explore the influence of key factors on the accuracy of these equations, such as the complexity of the track, the precision of the measurements, and the numerical methods used to calculate the length and area.
Analysis:
The IA could include graphical representation, derivatives, and integrals to draw conclusions about the accuracy of the parametric equations and their practical applications in the design and analysis of rollercoasters.
25.) Calculating the Volume of an Irregular Object using Displacement
Mathematics:
This topic involves an investigation into the use of displacement to calculate the volume of an irregular object, such as a rock, a sculpture, or a piece of driftwood.
Procedure:
The IA would involve measuring the volume of a liquid displaced by the object, and then using mathematical techniques to calculate the volume of the object. The research could also explore the influence of key factors on the accuracy of this method, such as the shape and size of the object, the precision of the measurements, and the effects of buoyancy and surface tension. The IA could include graphical representation, dimensional analysis, and error analysis to draw conclusions about the accuracy of this method and its practical applications in scientific and engineering contexts.
Analysis:
This topic could also involve comparing displacement-based methods with other volume calculation methods, such as integration and approximation, to evaluate their strengths and limitations.
26.) Modeling the Spread of an Infectious Disease using the SIR Model
Mathematics:
This topic involves an investigation into the use of the SIR model to simulate the spread of an infectious disease, such as influenza or COVID-19.
Procedure:
The IA would involve developing a mathematical model that describes the transmission dynamics of the disease, and then using simulation techniques to analyze the behavior of the model. The research could also explore the influence of key factors on the dynamics of the disease outbreak, such as the basic reproduction number, the effectiveness of control measures, and the duration of immunity.
Analysis:
The IA could include graphical representation, sensitivity analysis, and parameter estimation to draw conclusions about the effectiveness of the model and its practical applications in public health and policy contexts. This topic could also involve extending the SIR model to incorporate additional factors, such as age structure or spatial heterogeneity, to improve the accuracy of the simulations.
27.) Optimizing the Dimensions of a Cardboard Box for Minimum Material Cost
Mathematics:
This topic involves an investigation into the use of optimization techniques to determine the optimal dimensions of a cardboard box that minimizes material cost, while still satisfying specific constraints on volume or shape.
Procedure:
The IA would involve developing a mathematical model that describes the cost of manufacturing a cardboard box in terms of the dimensions of its sides, and then using optimization techniques to find the dimensions that minimize the cost. The research could also explore the influence of key factors on the solution, such as the size and strength of the cardboard sheets, the tolerance for waste material, and the restrictions on the shape of the box.
Analysis:
The IA could include graphical representation, derivatives, and linear programming to draw conclusions about the optimal solution and its practical applications in industry and manufacturing contexts. This topic could also involve extending the model to account for multiple variables, such as the thickness or type of the cardboard, or incorporating additional constraints, such as those related to shipping and storage.
28.) Investigating the Relationship between Student Attendance and Academic Performance
Mathematics:
This topic involves an investigation into the use of Pearson’s correlation coefficient to assess the relationship between student attendance and academic performance, using data from a sample of students.
Procedure:
The IA would involve collecting data on student attendance and academic grades, and then calculating the correlation coefficient to determine the strength and direction of the relationship. The research could also explore the influence of other factors, such as the number of missed classes, the type of course, and the difficulty level of the material, on the correlation.
Analysis:
The IA could include graphical representation, hypothesis testing, and significance testing to draw conclusions about the significance of the correlation and its practical applications in education and policy contexts. This topic could also involve comparing Pearson’s correlation coefficient with other measures of correlation, such as Spearman’s rank correlation coefficient, to evaluate their suitability for different types of data and research questions.
29.) Exploring the Golden Ratio in Art and Architecture
Mathematics:
This topic involves an investigation into the use of the golden ratio in art and architecture, and how mathematical techniques can be used to identify and analyze its presence in visual design.
Procedure:
The IA would involve researching the history and significance of the golden ratio in art and architecture, and then identifying examples of its use in a particular artwork or architectural structure. The research could also explore the mathematical properties of the golden ratio, such as its connection to the Fibonacci sequence and its relationships with other geometric figures.
Analysis:
The IA could include graphical representation, measurements, and calculations to demonstrate how the golden ratio is incorporated into the design, and to draw conclusions about its impact on visual aesthetics and composition. This topic could also involve investigating the use of the golden ratio in other fields, such as music or literature, to explore its applications beyond visual arts.
IB Tutoring Recent Blogs
Give our blog a read for anything you need
